What is it about fact fluency that is so challenging for some students? It’s not uncommon for students to lack automaticity with their addition, subtraction, multiplication, and division facts into middle school and beyond. Struggles with fact fluency sometimes accompany low achievement in math, but that is not always the case. Sometimes struggling math students know their facts. Sometimes high-achieving students do not know their facts.
I am reading John Tapper’s excellent new book entitled Solving for Why: Understanding, Assessing, and Teaching Students Who Struggle with Math. Tapper devotes a section of the book to students who face challenges in short term, long term and working memory. He weighs in on the fact fluency question there.
What students need to understand are underlying mathematics concepts. Multiplicative and proportional reasoning are, for example, critical to moving on from elementary mathematics. Fact retrieval certainly facilitates learning in these areas, but the inability to retrieve facts will not prevent students from reasoning at higher levels. Knowing math facts is important, but fact retrieval is to mathematics what spelling is to literacy: we want students to be proficient at the skill, but the skill is a small part of the overall picture. If a student is able to spell but cannot write a coherent essay, the spelling does them little good. The same is true with math facts. (p. 138)
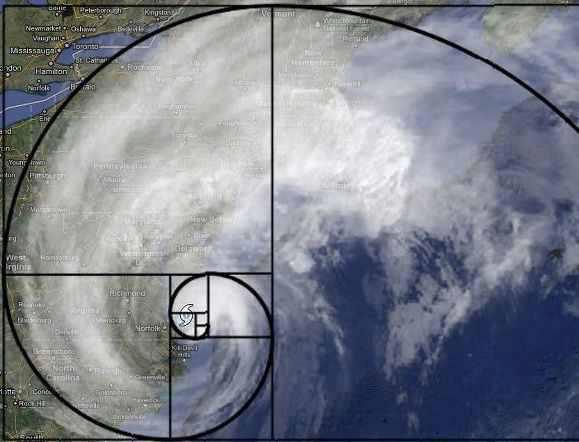
This is interesting for students, parents, and teachers to ponder. Try spending ten minutes a day or less studying math facts. Learn them in a way that reinforces conceptual understanding and is fun. Enjoy higher level, rich mathematics. Like the Fibonacci spiraling Hurricane Sandy picture above.

Alex Reutter, C.P. Smith Math Night Parent Extraordinaire, got me thinking about hexaflexagons. The other day, he mentioned that he thought kids would enjoy making them at Math Night. Hexaflexagons are folded paper hexagons that do a special flip. I decided I’d better try to figure these out ahead of time on a nice calm weekend at home.
I began by watching Vi Hart’s videos about hexaflexagons. Of course, Vi is fond of speeding things up, but I still thought I should be able to fold a strip of paper into equilateral triangles and make a hexaflexagon. Vi didn’t seem to be doing any measuring, like other websites recommend, so I was resistant to using a ruler. But after a bunch of mangled paper strips, I knew I needed a different strategy.
I found some pre-made, printable PDFs of hexaflexagon patterns. Some of the best are on a website called Aunt Annie’s crafts, on her Flexagons page. Print them, preferably in color. My brother and I were going to drive all the way down to Thetford, so I packed up some flexagon patterns, a scissor, and some double-sided tape for the trip.

I had plenty of time in the car to cut, tape, and fold several hexaflexagons, but I still didn’t know how to get them to do their special flip. I handed one to my brother while we stood around watching the Thetford cross country races, and he was able to figure it out.
These hexaflexagons are really cool. It’s worth trying one yourself. They were discovered by Arthur H. Stone in 1939, then popularized by Martin Gardner in his Scientific American column called “Mathematical Games” in 1956. Try pre-made patterns at first. I think starting students this way, then asking them how they might create their own if given a blank piece of paper, pencil, ruler, glue, and scissors, would be an excellent math activity, perfect for differentiation. We'll see how it goes at Math Night!





