Ada Lovelace

It is always good to hear about female mathematicians. Today, Google featured a graphic with the tag “Ada Lovelace’s 197th birthday”. Investigation revealed that Ada Lovelace was the daughter of the poet, Lord Byron, and lived in the 1800s.

A paragraph in the Washington Post caught my attention.

At the age of 17, Lovelace was among the first to grasp the importance of Babbage’s machines, Google noted. In her correspondence, as reported by New Scientist magazine, Lovelace said that “the Analytical Engine weaves algebraical patterns just as the Jacquard-loom weaves flowers and leaves.” She also noted that the Analytical Engine “does not occupy common ground with mere calculating machines” and had the potential to run complicated programs of its own.

Apparently, Lovelace wrote the first algorithm designed to be run by the Analytical Engine. Some say she should be considered the first computer programmer.

Here is another Lovelace quote to ponder (from Wikipedia:Ada Lovelace)

[The Analytical Engine] might act upon other things besides number, were objects found whose mutual fundamental relations could be expressed by those of the abstract science of operations, and which should be also susceptible of adaptations to the action of the operating notation and mechanism of the engine...

Supposing, for instance, that the fundamental relations of pitched sounds in the science of harmony and of musical composition were susceptible of such expression and adaptations, the engine might compose elaborate and scientific pieces of music of any degree of complexity or extent.[58]

The Wheel Shop

There’s a great website called Inside Mathematics. I learned about it at the Common Core Math sessions this year with Bob and Judi Laird, Sandi Stanhope, and Fran Huntoon, of the Vermont Mathematics Initiative.

Inside Mathematics is a treasure trove for teachers. It has problems of the month linked to Common Core standards. The best part about the problems is the fact that they begin with an easy level and progress to more difficult levels related to the same math and situation. It’s kind of like how the Bedtime Math website works.

Here’s one we tried today with Sandi Stanhope: The Wheel Shop. It starts innocently enough with Level A. There are 18 wheels in the shop that sells tricycles. How many tricycles are there in The Wheel Shop?

Then we went on. The Wheel Shop sells other kinds of vehicles. There are bicycles and go-carts in a different room of the shop. Each bicycle has only one seat and each go-cart has only one seat. There are a total of 21 seats and 54 wheels in that room. How many are bicycles and how many are go-carts?

Nick Mack had a lovely way to solve this one today at the workshop which involved doubling 21, then adding 2 wheels to bicycles until he had the right number of wheels. The bikes with the added wheels became the number of go-carts.

Nick said his second graders did a similar problem involving ducks and sheep. They drew a bunch of ducks then added 2 more legs to several of them in order to “turn them into sheep”. He said the sheep that began as ducks were fairly ugly, but the math worked out.

Today Sandi said, "Math should be hard. Let kids figure it out." 

Here is the Level C problem:
Three months later some vehicles have sold and new models have been brought into the Wheel Shop. Now, there are a different number of bicycles, tandem bicycles, and tricycles in the shop. There are a total of 135 seats, 118 front handlebars (that steer the bike), and 269 wheels. How many bicycles, tandem bicycles and tricycles are there in the Wheel Shop?

Today Nick started making a spreadsheet for this one and I did something similar tonight. My spreadsheet had columns for handlebars, seats, and wheels and did some multiplication and addition with formulas to get the totals. I guess-and-checked my way as the spreadsheet did the calculation. Setting this up took about 10 minutes, but my husband, Jim, had already gotten the answer and was eating muffins with the kids in the kitchen by the time I figured it out.

Jim had written his solution on an index card. He explained it as follows:

There are 118 total bikes (because there were 118 total front handlebars).
Multiply that by 2 and get 236 (so there must be 236 wheels).
But there are 269 wheels, so I subtracted. 269-236=33. There are 33 tricycles.
There were 135 seats but only 118 steering handlebars, so I subtracted. 135-118=17.
There must be 17 tandems and 33 tricycles. This left 68 regular bicycles because I subtracted 17 and 33 from 118 to get 68.



Enjoy the problems of the day! We did.

Math Warmups

Lately, we are experimenting with math warmups in elementary school classes. Today, I tried one on a fourth grade class. I think someone else tried the same warmup in a fifth grade class, so I will have to ask them how it went.

Math warmups take just 5 to 10 minutes at the start of math class. For maximum effect, they are done every day or almost every day. With math warmups, it is possible to teach specific skills related to number sense. Lots of math gurus talk about these, including Sandi Stanhope and Bob Laird from the Vermont Mathematics Initiative, Marilyn Burns, Cathy Fosnot, etc. etc.

Here’s the one from today:

4 x 8 =
8 x 4 =

8 x 3 =
4 x 6 =
2 x 6 =

1 x 6 =
½ x 6 =
¼ x 6 =

I asked the students to solve these mentally and write the answers. I didn’t want anyone to struggle, so, if they didn’t know it, I just told them or had another student tell them the answer. I encouraged students to use what they knew from the previous equations to help them find answers without the usual calculation.

When asked what they noticed, students were able to share that they saw the commutative property in action (without using that vocabulary word) and could see halving and doubling relationships.

For example, 8 x 3 and 4 x 6 both equal 24, and that 4 is half of 8 but 6 is double 3. When you double something then halve it, you are back to where you started.

Getting down to the series of _ x 6 was interesting. Students noticed the pattern of the 6 staying the same while the other factor kept getting reduced by half. By the time they got down to the fractions, they were able to use their knowledge of the properties of multiplication to help them through a potentially difficult task. These students haven’t yet studied much about multiplication of fractions, but were able to do it easily.

I am thinking in the future it might be fun to try a number times ¼ and let students work back up to one through doubling if necessary to assist them in solving the equation without an algorithm.

Is Welding a STEM job?

New York Public Library Digital Gallery

Victor Prussack, Burlington’s Coordinator of Magnet Schools, found this Thomas Friedman article in the New York Times over the weekend and was kind enough to send it to me.

The piece begins with a profile of a sheet metal business owner from Minnesota named Traci Tapani. She can’t find enough skilled welders.

“Many years ago, people learned to weld in a high school shop class or in a family business or farm, and they came up through the ranks and capped out at a certain skill level. They did not know the science behind welding,” so could not meet the new standards of the U.S. military and aerospace industry.

“They could make beautiful welds,” she said, “but they did not understand metallurgy, modern cleaning and brushing techniques” and how different metals and gases, pressures and temperatures had to be combined.

Welding “is a $20-an-hour job with health care, paid vacations and full benefits,” said Tapani, but “you have to have science and math. I can’t think of any job in my sheet metal fabrication company where math is not important. If you work in a manufacturing facility, you use math every day; you need to compute angles and understand what happens to a piece of metal when it’s bent to a certain angle.”

Who knew? Welding is now a STEM job — that is, a job that requires knowledge of science, technology, engineering and math.

I was reading this article to my kids tonight and it occurred to me that even today’s doctors and lawyers - always regarded as professions for the highly educated - have undergone a STEM transplant. Doctors of today have unprecedented levels of information access and global collaboration, and are using online medical records, sophisticated medical imaging technology, gene therapies, remote robotic surgery, and more. Lawyers might have to deal with things that didn’t exist not long ago like various forms of DNA and IT evidence.

If you wanted to be an administrative assistant or a librarian, you used to be able to use a Rolodex and the card catalog. Now these jobs are all about technology.

New York Public Library Digital Gallery

The Struggle

John J. Flynn Elementary Principal Graham Clarke alerted me to a very nice piece on NPR today. It’s called Struggle for Smarts? How Eastern and Western Cultures Tackle Learning.

A researcher was studying students in a Japanese school, and ended up observing a fourth grade math class.

"The teacher was trying to teach the class how to draw three-dimensional cubes on paper," Stigler explains, "and one kid was just totally having trouble with it. His cube looked all cockeyed, so the teacher said to him, 'Why don't you go put yours on the board?' So right there I thought, 'That's interesting! He took the one who can't do it and told him to go and put it on the board.' "

Have a listen. It’s only 8 minutes.

Fact Fluency

What is it about fact fluency that is so challenging for some students? It’s not uncommon for students to lack automaticity with their addition, subtraction, multiplication, and division facts into middle school and beyond. Struggles with fact fluency sometimes accompany low achievement in math, but that is not always the case. Sometimes struggling math students know their facts. Sometimes high-achieving students do not know their facts.

I am reading John Tapper’s excellent new book entitled Solving for Why: Understanding, Assessing, and Teaching Students Who Struggle with Math.  Tapper devotes a section of the book to students who face challenges in short term, long term and working memory. He weighs in on the fact fluency question there.

What students need to understand are underlying mathematics concepts. Multiplicative and proportional reasoning are, for example, critical to moving on from elementary mathematics. Fact retrieval certainly facilitates learning in these areas, but the inability to retrieve facts will not prevent students from reasoning at higher levels. Knowing math facts is important, but fact retrieval is to mathematics what spelling is to literacy: we want students to be proficient at the skill, but the skill is a small part of the overall picture. If a student is able to spell but cannot write a coherent essay, the spelling does them little good. The same is true with math facts. (p. 138)

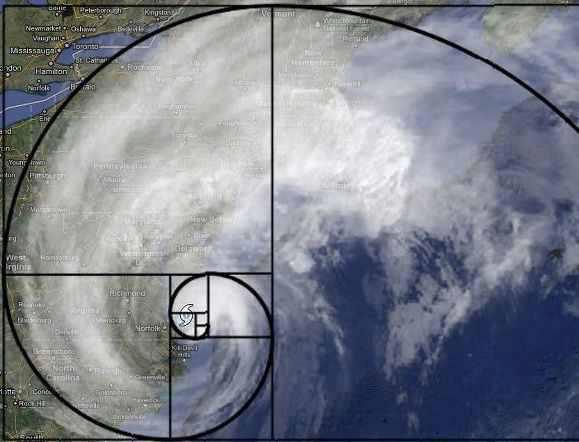
This is interesting for students, parents, and teachers to ponder. Try spending ten minutes a day or less studying math facts. Learn them in a way that reinforces conceptual understanding and is fun. Enjoy higher level, rich mathematics. Like the Fibonacci spiraling Hurricane Sandy picture above.

Hexaflexagons

Alex Reutter, C.P. Smith Math Night Parent Extraordinaire, got me thinking about hexaflexagons. The other day, he mentioned that he thought kids would enjoy making them at Math Night. Hexaflexagons are folded paper hexagons that do a special flip. I decided I’d better try to figure these out ahead of time on a nice calm weekend at home.

I began by watching Vi Hart’s videos about hexaflexagons. Of course, Vi is fond of speeding things up, but I still thought I should be able to fold a strip of paper into equilateral triangles and make a hexaflexagon. Vi didn’t seem to be doing any measuring, like other websites recommend, so I was resistant to using a ruler. But after a bunch of mangled paper strips, I knew I needed a different strategy.

I found some pre-made, printable PDFs of hexaflexagon patterns. Some of the best are on a website called Aunt Annie’s crafts, on her Flexagons page. Print them, preferably in color. My brother and I were going to drive all the way down to Thetford, so I packed up some flexagon patterns, a scissor, and some double-sided tape for the trip.

I had plenty of time in the car to cut, tape, and fold several hexaflexagons, but I still didn’t know how to get them to do their special flip. I handed one to my brother while we stood around watching the Thetford cross country races, and he was able to figure it out.

These hexaflexagons are really cool. It’s worth trying one yourself. They were discovered by Arthur H. Stone in 1939, then popularized by Martin Gardner in his Scientific American column called “Mathematical Games” in 1956. Try pre-made patterns at first. I think starting students this way, then asking them how they might create their own if given a blank piece of paper, pencil, ruler, glue, and scissors, would be an excellent math activity, perfect for differentiation. We'll see how it goes at Math Night!

There is a free iPad app from IBM called Minds of Modern Mathematics. It is a beautiful timeline of the history of mathematics and world events, and there are 9 vintage videos to watch as well. Charles and Ray Eames, a famous, husband-and-wife design team created the original interactive mathematics exhibit back in 1961, which provided the content for this app. The Eameses films are wonderful, simple, short. I enjoyed Something About Functions, and the non-verbal Exponents.

I also happened upon a great blog called MathMunch. MathMunch calls itself “a weekly digest of the mathematical internet”. They posted something about Minds of Modern Mathematics, and have a fabulous page of math videos. If you miss the old Schoolhouse Rock videos and you know a young person who could use some work on counting by 5s, MathMunch has the link. Their video collection is exhaustive, and includes videos about solving a Rubik’s Cube, paperfolding, Pi, M.C. Escher, and more.

Teachers’ Expectations

When I arrived at work last week, several people were excitedly talking about the NPR piece they’d heard on the radio during their drive in. Here is a link to the Morning Edition show, Teachers’ Expectations Can Influence How Students Perform, which aired September 17.

I recommend listening to the audio, but you can also read the transcript. Back in 1964, Harvard professor Robert Rosenthal began studying how teachers’ expectations influence student achievement.

[Rosenthal] found that expectations affect teachers' moment-to-moment interactions with the children they teach in a thousand almost invisible ways. Teachers give the students that they expect to succeed more time to answer questions, more specific feedback, and more approval: They consistently touch, nod and smile at those kids more.

"It's not magic, it's not mental telepathy," Rosenthal says. "It's very likely these thousands of different ways of treating people in small ways every day."

It is difficult to truly change our beliefs, but there is a way. Recent studies have shown that teachers who actively worked on their teaching through videotape analysis and targeted work with coaches in their classrooms to change their behavior also experienced a significant shift their beliefs about students.

What's Math Got to Do with It?

A man is on a diet and goes into a shop to buy some ham slices. He is given 3 slices which together weight ⅓ of a pound, but his diet says that he is only allowed to eat ¼ of a pound. How much of the 3 slices he bought can he eat while staying true to his diet?

There is a lovely book in the John J. Flynn Parent Resource Center these days. It’s called What’s Math Got to Do with It? How parents and teachers can help children learn to love their least favorite subject, by Jo Boaler. The book was published in 2009, but I have only recently discovered it.

Boaler includes many more problem-solvers like the one above. Don’t worry if you don’t know a formula to figure out how many turkey slices the man can eat. Start drawing pictures and think about what a whole pound would look like. Go slow and use your intuition. Says Boaler, “Children begin school as natural problem solvers and many studies have shown that students are better at solving problems before they attend math classes.”

…People don’t like mathematics because of the way it is misrepresented in school. The math that millions of Americans experience in school is an impoverished version of the subject and it bears little resemblance to the mathematics of life or work or even the mathematics in which mathematicians engage.

In addition to the prompt I shared in my last post “What do you think you should try next?”, Boaler shares more good prompts to use with children:

• How did you think about the problem?
• What was the first step?
• What did you do next?
• Why did you do it that way?
• Can you think of a different way to do the problem?
• How do the two ways relate?
• What could you change about the problem to make it easier or simpler?

Enjoy.

Teach your children well

 

Here’s a nice article from the Wall Street Journal for parents, with an accompanying video. 

This short piece hits the important points when it comes to math and parenting. 

The one thing I would add is my favorite question for my daughter when she asks for help with her algebra homework: “What do you think you should try next?”

Mindset

Charles Coiner, 1961, from Smithsonian American Art Museum

I am a huge fan of Carol Dweck’s work on people’s beliefs about the nature of intelligence. A student’s mindset has a measurable impact on his or her success in math, among other things.

We praised the children in one group for their intelligence, telling them, “Wow, that’s a really good score. You must be smart at this.” We praised the children in the other group for their effort: “Wow, that’s a really good score. You must have worked really hard.” That’s all we did, but the results were dramatic.

Doesn’t that statement make you want to read more? Here is a condensed version of Dweck’s research (you can read the book, Mindset, and find more articles here).

And here is a student-friendly version of the article. Perfect non-fiction reading for fourth and fifth graders!

The Necessity of Algebra

On Sunday, the New York Times published a piece by Andrew Hacker with the provocative title “Is Algebra Necessary?”. Several people have emailed me the link, so it seems like it has garnered some attention. It is worth reading.

While I disagree with most of Hacker’s anti-advanced-math argument, the statistics about the barrier students face when they are not proficient in high school math is important to consider. Failure in math does profoundly affect a young person’s options when it comes to college and career choices. How many people do you know whose math ability was a significant factor in their choice of college major and career? Just the other day, a friend admitted that she’d always dreamed of being a doctor, but she wasn’t good at math so she went into journalism instead.

Rather than lowering the bar for students, we should look at how we can improve mathematics education. Algebra is about abstraction, which is one of the most fundamental ideas across disciplines. When I was a software engineer, much of my day-to-day work was about designing abstract functions with variables to hold unknowns. It was about finding clean, elegant solutions to complex problems through thought, collaboration, trial and error. We conjectured, tested, proved. I couldn’t have learned concrete “job skills” ahead of time. I had to keep learning, inventing and working with big ideas. Great algebra and other math classes are excellent preparation for students, as are great science, literature, writing, and history classes.

Hacker’s article prompted many thoughtful responses. I’ve collected some here along with excerpts for your reading pleasure. I’ll end with a nice video of Paul Lockhart talking about the wonderfulness of math.

The New York Times Letters to the Editor: A National Conversation on Math
Andrew Hacker is right: most students will never need to use algebra. Many will struggle to learn it nonetheless. But the answer is not to let students quit as soon as they begin to struggle.

I myself hated mathematics for many years. Through algebra, geometry and trigonometry, I cursed a system that compelled me to take such “useless” courses. Eventually, I was required to take calculus, the most dreaded of all math courses. I prepared for the worst.

It came as a surprise, then, that I quickly found myself enjoying the class. The reason was that I had finally encountered a talented math teacher with a passion for the subject. His passion proved infectious, and now, a year later, I’m looking to study mathematical biology at an Ivy League university.

It’s an outcome I would have never predicted just a few years ago. It could have never happened if I had been allowed to quit when I first struggled with math.

ZACHARY MILLER
Kitty Hawk, N.C., July 29, 2012

Motherlode: Adventures in Parenting by Jessica Lahey
...I know precisely where I lost my battle with math, the moment I was informed clearly and unequivocally that I simply wasn’t “a math person.” My seventh-grade math teacher, an otherwise lovely man, called each of his students up to his desk one by one in order to write a “1” (for the honors track) or “2” (for the standard track) on the school’s official math placement forms. As I watched from over his hunched and courduroyed shoulder, he wrote a beautiful, decisive and neat “1” on my form.

There it was, in permanent ink. I was good at math.

“Jess, could you come back up here for a minute?” he asked as I floated back to my seat.
He reclaimed my form, and carefully overlaid that beautiful “1” with a dark, clumsy “2,” pressing hard with his black pen in order to make sure the ink obliterated any evidence of his indecision.

And from then on, I wasn’t good at math anymore....

Scientific American: Abandoning Algebra is Not the Answer by Evelyn Lamb
Mathematicians are recruited by hedge funds, consulting firms, and technology companies not because they already know how to balance portfolios, what the best corporate strategies are, or how to optimize user interfaces, but because their mathematics degrees indicate experience and acuity at problem solving. It’s easier for companies to teach someone with a strong mathematics background how to do their specific work than to teach someone who knows the company business how to solve problems. And, like it or not, algebra is one of the first places students start to learn these problem solving skills....

Math education needs to improve, but if illiteracy were on the rise, I don’t think we’d be talking about eliminating reading from the curriculum.

Rob Knop
Algebra is fundamental to nearly all of "higher math". Even if you want to do more than the most basic of things with statistics, you need to know some algebra. To give up on that would be right on par with the giving up on the teaching of history as anything other than memorizing the occasional date, and to give up on the teaching of English literature as anything other than being able to read a short document for simple surface content and to put together a simple declarative sentence. If you want people to be educated beyond elementary school and beyond "job training", then algebra is one of the intellectual foundations of our civilization that simply cannot be neglected.

Harvard University Press Blog: Is Mathematics Not Beautiful?
For all his focus on the pain and fear of mathematics, Hacker has little to say about its beauty. He does suggest that we should treat mathematics as a liberal art, “making it as accessible and welcoming as sculpture or ballet,” but in the service of rationalizing its marginalization rather than encouraging its embrace.

What must be and what can't be

Have you heard of KenKen puzzles? They are logic puzzles like Sudoku but with an extra element of math calculation. They are great challengers for people of all ages and abilities, since there is such a great range of levels of difficulty. I’ve given them to students, teachers, and friends. 


My dad first introduced them to me. He must have discovered them when they showed up in the New York Times on the same page as the crossword puzzle. He does them in pen, with many cross-outs. The answers to the previous day's puzzles are always there, but they are unnecessary. You know when you've got it right.

I have figured out how to do KenKen puzzles and can do an OK job, especially with the easier puzzles. I want to be better than I am now, so I know I need practice. My dad and mom are visiting me in Vermont this week, so I decided to see if I could get some help with my KenKen chops.

Here is my dad’s advice to me: “First you look at the arithmetic, put in some candidate numbers. Then you start looking for what must to be and what can’t be. Remember to see the whole puzzle even as you work on the pieces. Don’t quit. Keep looking for what must be and what can’t be. If you look at it a little bit you will see it. If you get really stuck, go pour yourself a glass of Pinot Noir or something and the answers will become evident.”

Dad is all about creative problem-solving perseverance, which means he is down with the Math Common Core without knowing it. I was stuck on the harder of the two New York Times puzzles today but he freed me with one little tip. I filled in the rest of the numbers almost as fast as I could write. The tip went something like, “This has to be a 5 and that can’t be a 5 so it must be a...”.

KenKen puzzles are great for young mathematicians. My youngest KenKen student was in first grade. I think most first graders are too young, but she nailed it. I like giving out packets that start with the easier 3x3 grids and are limited to addition and subtraction, and then progress to larger grids with multiplication and division. Most students can quickly find a just-right puzzle and dive in.

The best part of this story is that the marvelous Phia S., a student entering fifth grade, loves KenKens so much she decided to write her own. Here is a photo of the treasured book of KenKen’s given to me by Phia. 

Phia's amazing KenKen book

So, enjoy your summer and take your KenKens (and Pinot if you are of age) to the lake or wherever you might be relaxing. The New York Times publishes two a day on the crossword page (they always include the directions) and more online. Cheers!

Visit from a future pilot

Kim Kast, amazing afterschool math teacher at John J. Flynn Elementary, contributed this piece. Thank you, Kim!
 
Last week, third grade math mania students enjoyed a visit from a special guest, University of Vermont student Sylvia Stevens-Goodnight. Sylvia just completed her sophomore year of study in the UVM College of Engineering and Mathematical Sciences. Her concentration is mechanical engineering, and she told the students she is one of only seven women in her program; she encouraged the students in their studies of math, and wanted to especially encourage girls to consider pursuing a career involving the study of math and science. Sylvia described some career-related experiences, such as trying on a pilot's suit, sitting at the controls of a jet plane, and even being ejected from the seat of the plane. Sylvia explained to the students that engineers sometimes design new products, invent new machines, and innovate new ideas. The students were surprised to learn that engineers could even design video games.

All of the students had many questions about engineering, and of course, about college life in general. The third graders couldn't believe that a student could have seconds, or even thirds, on ice cream for dessert... every night! Sylvia assured them (speaking from experience) that after a few days of all-you-can-eat ice cream, they would know why their mothers wisely told them, "Eat your vegetables!"

Music for the Eyes

Joe Garofalo, C.P. Smith music teacher and captain in the Lyric Theater’s recent production of Titanic, kindly shares his room with me. Often, before the students arrive in the morning, we find ourselves discussing the math-music connection and how we might help bring this to life for students. Last week, Joe showed me some animated music he’d discovered. I had a hard time tearing myself away. See for yourself. Here is Johann Sebastian Bach’s Toccata and Fugue in D Minor  by a company called Musanim. Musanim says it will send a free DVD of these videos to public libraries and schools. We’ve got ours on order.

Scale of the Universe

 

What do Gomez’s Hamburger, Palm Jebel Ali and Mimivirus have in common? They are all found in Scale of the Universe 2 at 2.5 x 1015 meters, 8 x 103 meters, and 4 x 10-7meters, respectively. Scale of the Universe 2 is a cool, interactive website which goes nicely with my earlier blog post about the Powers of Ten lesson and video. Visitors can zoom all the way down to teeny Quantum Foam and then all the way out to the observable universe and the Hubble Deep Field.

Each object has a brief description, so you will not be left wondering what Palm Jebel Ali is (the largest human-made island) and you’ll know Gomez’s Hamburger is a heavenly body, not a menu item. There are commonplace entries like a Boeing 747 and a sunflower, too.

Students can use this site to explore relative magnitude even if they are not yet ready to learn about exponents in math. Author Istvan Banyai has written a wonderful book called Zoom, which does something similar in low-tech. Students might try to create their own book in this format.

John J. Flynn library super-star Corey Wallace brought Scale of the Universe to my attention. This is just another example of how my own universe is constantly expanding with the help of my talented and enthusiastic colleagues.

 

A Pickle

There are some interesting new websites out there. One is called Math Pickle. Shannon Walters, C.P. Smith Library Guru, alerted me to this one. So far, I like most of what I’ve seen on this site, although the layout is a bit clunky. Dr. Gordon Hamilton presents activities for students based on math problems dating back several decades to mathematicians like Issai Schur and Lothar Collatz. His decision to put young Vi Hart on the same page as some of the older mathematics greats is a good sign. Math activities are presented via video, and include footage of real students. I’m less impressed with Dr. Hamilton’s philosophizing about the way math should be taught in a video entitled “Let’s Abolish Elementary Mathematics”, even though I agree with most of what he is saying.

I like the Graceful Tree Conjecture as a means of practicing subtraction with third graders and the Collatz Conjecture for fourth graders. These are captivating problem-solving activities which are an excellent way to encourage students to work together, discuss different strategies, practice skills, check their work, and have fun. I would also try the magic cauldrons addition game for second graders. Teachers will find Math Pickle a useful site for finding new activities for math class and getting a thorough explanation of how to use them.